COMSOL has the best multiphysical simulation capabilities in my experience. Technical support from Elisa at TECHNIC as well as the engineers at COMSOL has been great.
COMSOL is an important part of our research in plasma physics. We use it in the design of plasma systems and it helps us to obtain a greater understanding of the underlying physics. We have always valued the quick support from TECHNIC and COMSOL and it has been a pleasure to work with them.
Comsol has become a valuable part of our design and decision making process. The exceptional flexibility and access to the physics and solvers in Comsol has allowed us to have deeper understanding on thermomechanical solutions. Technic and Comsol have always been quick and helpful to resolve any issues and provide helpful advice on their products.
At Scion we use COMSOL Multiphysics to understand energy processes, such as the interplay of non-linear solid mechanics and heat & mass transfer during biomass compaction, to design new or more efficient processes.
We use COMSOL Multiphysics to design the customised muffler. With it, we can simulate the insertion loss at different spectrum with different muffler designs.
When structures are subjected to repeated loading and unloading due to material fatigue, they can fail at loads below the static limit. A virtual fatigue analysis can be performed in the COMSOL Multiphysics environment with the Fatigue Module, an add-on to the Structural Mechanics Module, which contains a collection of fatigue models. The applicability of each model depends on factors such as material and loading type.
The classical stress- and strain-life methods relate a stress or strain amplitude to a fatigue lifetime. Together with the stress-based and the strain-based critical plane methods, you can evaluate the high-cycle and low-cycle fatigue regime. In applications involving nonlinear materials, you can use energy-based methods or Coffin-Manson type models to simulate thermal fatigue. When dealing with variable loads, the accumulated damage can be calculated from the load history and the fatigue limit.
The fatigue load cycle can be simulated in solid bodies, plates, shells, multibodies, applications involving thermal stress and deformation, and even on piezoelectric devices. In order to improve computational efficiency when dealing with subsurface or surface initiated fatigue, a fatigue evaluation can be performed on domains, boundaries, lines, and in points.
Low-cycle fatigue resulting from plastic deformation near a hole showing the logarithm of lifetime in terms of number of cycles together with a stress-strain curve for the first few load cycles.
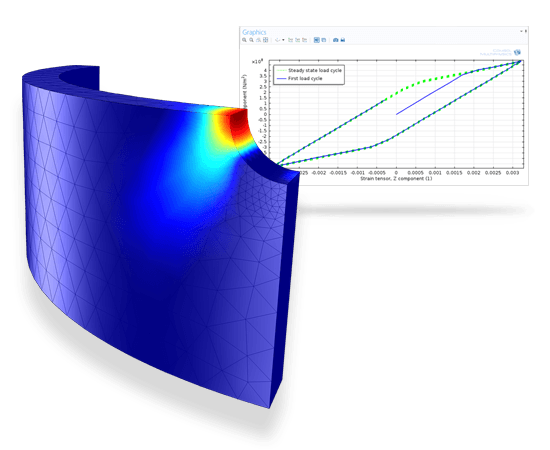
When analysing fatigue through classical methods, the stress or the strain amplitude relates to the fatigue lifetime via a fatigue curve. The stress-life and the strain-life models provide a collection of methods where the fatigue curve can be defined in a variety of ways. These models are suitable for proportional loading when, for example, a single load oscillates between two values. You can address the high-cycle fatigue with the stress-life models containing the classical S-N curve, Basquin model, and an approximate S-N curve. You can make low-cycle fatigue prediction using the strain-life models containing the E-N curve, Coffin-Manson, and Combined Basquin and Coffin-Manson model.
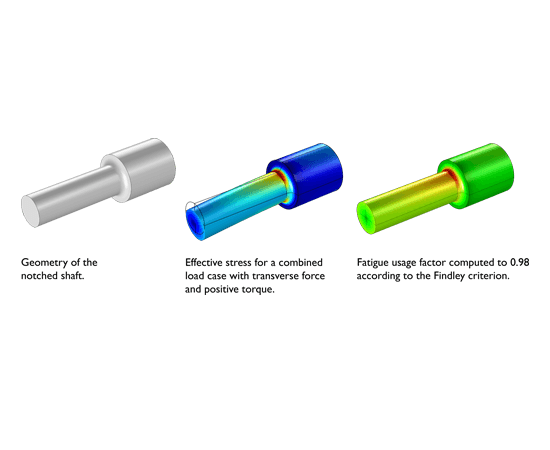
Critical plane models search for a plane that is most favourable for crack initiation and propagation where fatigue will take place. These are available in the Fatigue Module for both the stress- and strain-based models. In the high-cycle fatigue domain, where plasticity is very limited, stress-based models are typically used. In the Fatigue Module, they are calculated through the Findley, Normal stress, and Matake criteria, which calculate the fatigue usage factor that is compared to the fatigue limit.
Strain-based models evaluate strains or combinations of strains and stresses when defining a critical plane. Once the critical plane is identified, they predict the number of cycles to failure. The Fatigue Module features the Smith-Watson-Topper (SWT), Fatemi-Socie, and Wang-Brown models. These models are usually used in low-cycle fatigue where the strains are large. The Neuber's rule and the Hoffmann-Seeger method are available to approximate the effect of plasticity in a quick linear-elastic simulation. It is also possible to consider a full elastoplastic fatigue cycle when using the Nonlinear Structural Materials Module.
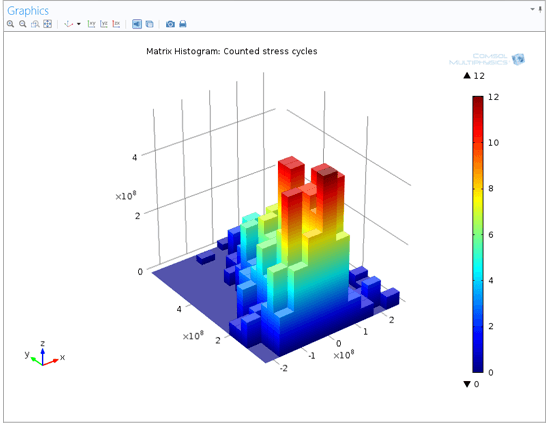
The Fatigue Module calculates the number of cycles until failure as well as the fatigue usage factor. In cumulative damage simulations, the stress distribution of the applied random load can be shown together with the relative usage factor. This simulation displays contributions of a specific fatigue load to the overall fatigue usage factor, which in this case is seen as damage. The stress distribution is presented as a function of the stress amplitude and the mean stress.
Random loads introduce a variety of stresses with different magnitude into a structure. In the Fatigue Module, the Cumulative Damage analysis not only identifies the overall trends in the stress history, but also calculates the accumulated damage from each of them. The stress history can be evaluated through the principal stress or von Mises stress, with a sign determined by principal or hydrostatic stress. The load history is then processed with the Rainflow counting algorithm, and the damage is calculated using the Palmgren-Miner linear damage rule. The effect of the R-value is incorporated through the limiting S-N curve.
When the number of load events is large in a random load analysis, simulation of the load cycle is time-consuming. This can be greatly reduced if the nonlinear effects are not present in the simulation. In that case, the stress cycle can be prescribed with help of superposition, which is selectable in a Cumulative Damage analysis. Utilisation of this technique not only saves computational time, but also greatly reduces the size of the model that needs to be stored for fatigue evaluation.
Material expansions or contractions due to temperature change introduce stress concentrations and strain accumulations that can lead to failure. The Fatigue Module provides a number of tools to address this through thermal fatigue modelling. The thermal load cycle can be simulated utilising the Thermal Stress and the Joule Heating and Thermal Expansion physics interfaces. Thermal fatigue failure can be evaluated using several fatigue models. For nonlinear materials, this includes the Coffin-Manson model and the energy-based Morrow and Darveaux relations. In addition to the available options for inelastic strains or dissipated energies, the fatigue evaluation models can also be modified by the user to evaluate strain or energy expressions when calculating fatigue.
Vibration fatigue is an evaluation method based on a frequency-domain analysis making results available for a number of frequencies. It is used for predicting the results from a common type of experiment where a structure is subjected to an excitation with increasing frequency, sometimes called a sine sweep analysis. The following settings are available for vibration fatigue analyses:
In order to fully evaluate whether or not the COMSOL Multiphysics® software will meet your requirements, you need to contact us. By talking to one of our sales representatives, you will get personalised recommendations and fully documented examples to help you get the most out of your evaluation and guide you to choose the best license option to suit your needs.
Fill in your contact details and any specific comments or questions, and submit. You will receive a response from a sales representative within one business day.
Tel: +61 (03) 6224 8690Email: info@technic.com.auConnect on LinkedInGet Your Free TrialCopyright © 2021 Technic Pty Ltd. All Rights Reserved. See our Privacy Policy. Site protected by reCAPTCHA - Privacy | Terms. Website by Gloo.
